These problems are part of the homework due on Monday, July 28.

1. Determine the largest integer $n$ that can’t be written as $5s+7t$, where $s$ and $t$ are nonnegative integers. Prove your answer, i.e., show that your $n$ can’t be written in this form and show that all larger integers can be written in this form.
2. How many paths are there from point A to point B in the grid below, if we can only travel along the lines going north (up) and east (right)? Be sure to notice the “gap” in the grid.
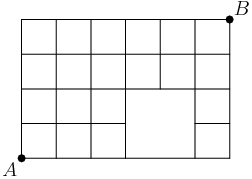
3. Determine how many ways 8 indistinguishable objects can be distributed to 4 indistinguishable boxes. List the ways.
4. Determine how many ways 8 distinguishable objects can be distributed to 4 indistinguishable boxes. Hint: Solve problem 3 first.